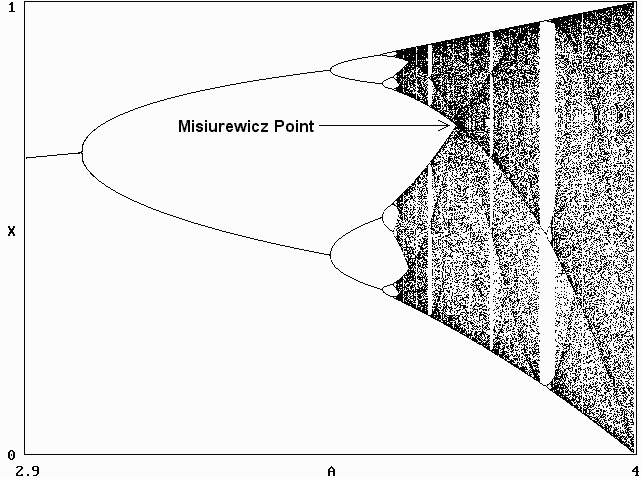
The exact value was determined by zooming into the corresponding region of the (A, X) diagram by a factor on the order of 1015 with nine iterations starting with an initial condition of X0 = 0.5, and the result is shown below:
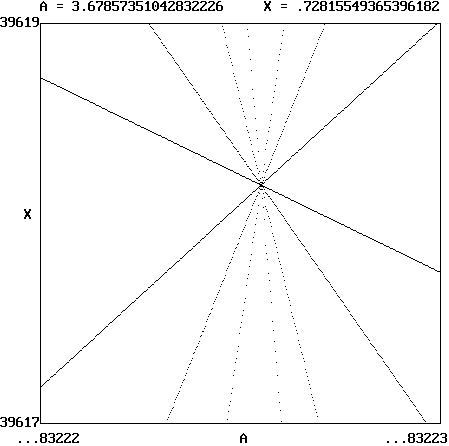
The calculated Misiurewicz point is A = 3.67857351042832226... at X = 0.728155493653961819...
According to Daniel Stoffer of the Mathematics Department at ETH-Zurich, A satisfies the equation A3 - 2A2 - 4A - 8 = 0, and X follows from X = 1 - 1/A. He also points out that the Misiurewicz point has the following properties:
- For any A less than or equal to the Misiurewicz point, the logistic map admits no periodic point of odd period except for a fixed point. For any A greater than the Misiurewicz point, the logistic map admits periodic points of odd period > 1. For A > 3.628 but less than or equal to the Misiurewicz point, the logistic map admits periodic points of any even period.
- If A is less than or
equal to the Misiurewicz point, then the logistic map does not admit a
snap-back repellor. (For the notion of a snap-back repellor, see
F. Marotto, Snap-back repellors
imply chaos in Rn, J. Math. Anal. Appl. (1978).)