Chaotic Dynamics on Large Networks
J. C. Sprott
Departments of Physics, University
of Wisconsin, Madison, WI 53706, USA
Received 30 March 2008; accepted 27 May 2008; published online 30 June
2008
ABSTRACT
Many systems in nature are governed by a large number of agents that
interact nonlinearly through complex feedback loops. When the networks
are sufficiently large and interconnected, they typically exhibit
self-organization and chaos. This paper examines the prevalence and
degree of chaos on large unweighted recurrent networks of ordinary
differential equations with sigmoidal nonlinearities and unit coupling.
The largest Lyapunov exponent is used as the signature and measure of
the chaos, and the study includes the effects of damping, asymmetries
in the distribution of coupling strengths, network symmetry, and
sparseness of connections. Minimum conditions and optimal network
architectures are determined for the existence of chaos. The results
have implications for the design of social and other networks in the
real world in which weak chaos is deemed desirable or as a way of
understanding why certain networks might exist on “the edge of chaos.”
Ref: J. C. Sprott,
Chaos 18, 023135-1 - 023135-9
(2008)
The complete paper is available
in PDF format.
Return to Sprott's Books and Publications.
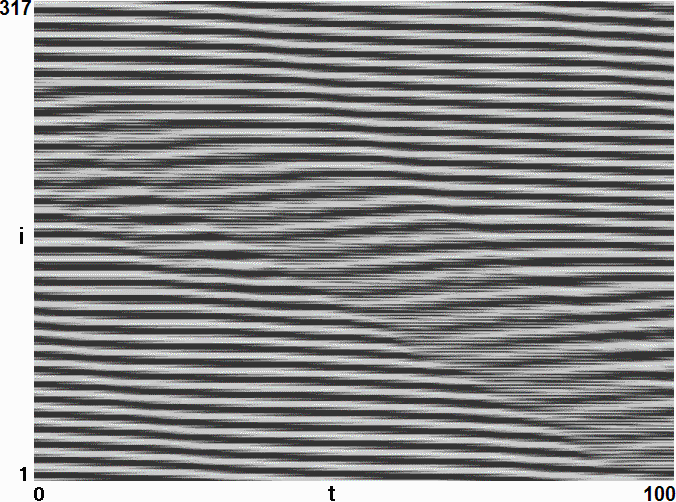
Fig. 1.
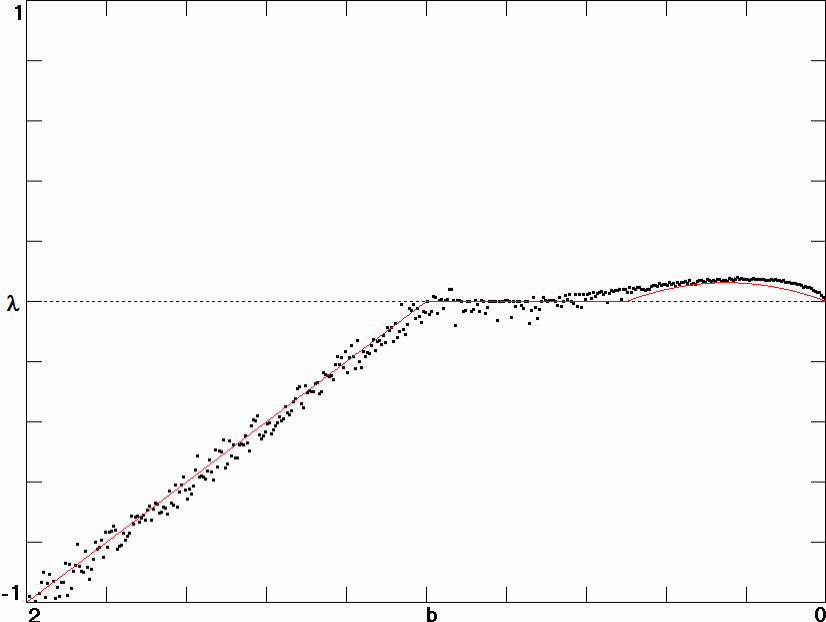
Fig. 2.

Fig. 3.
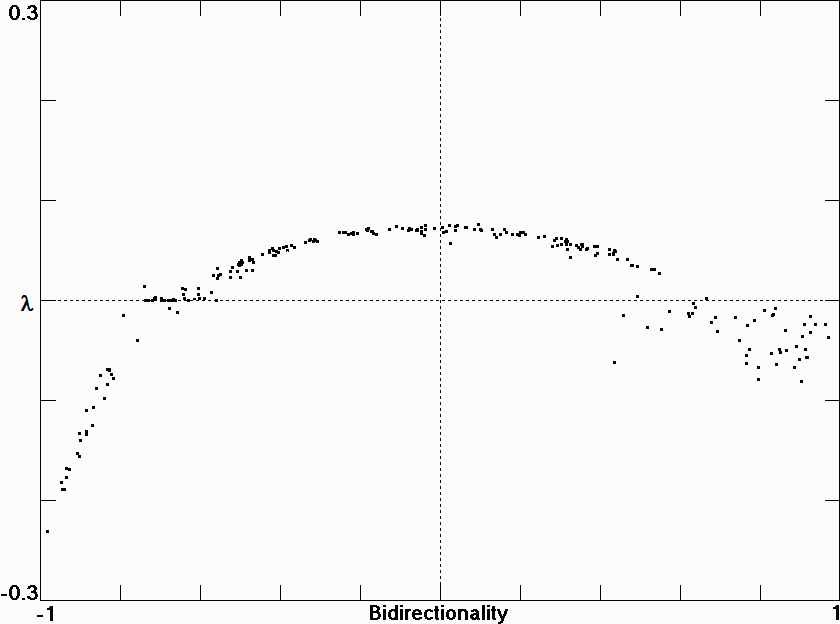
Fig. 4.
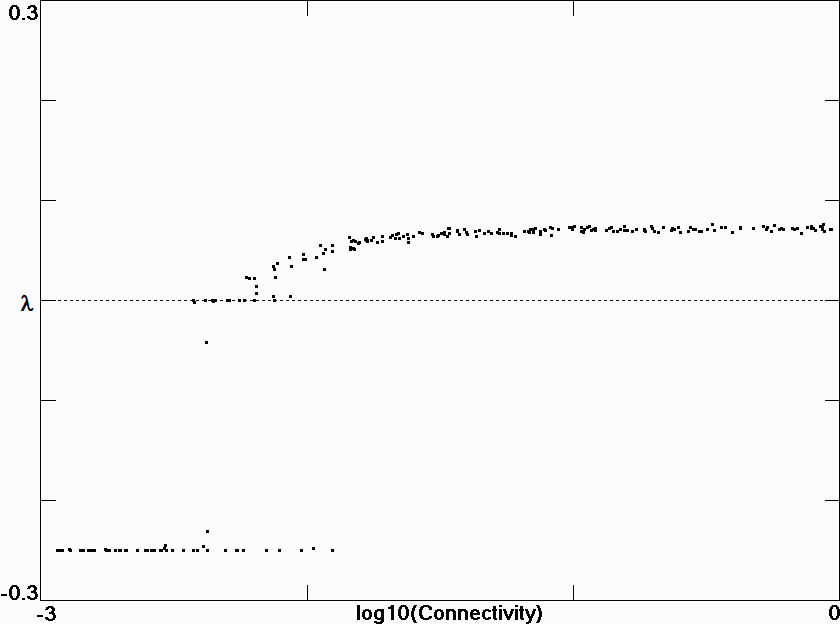
Fig. 5.
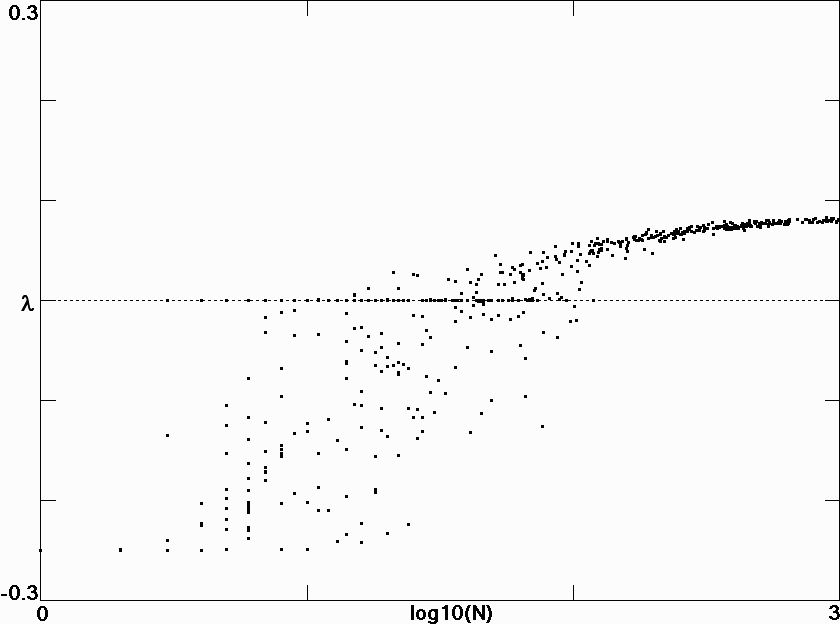
Fig. 6.
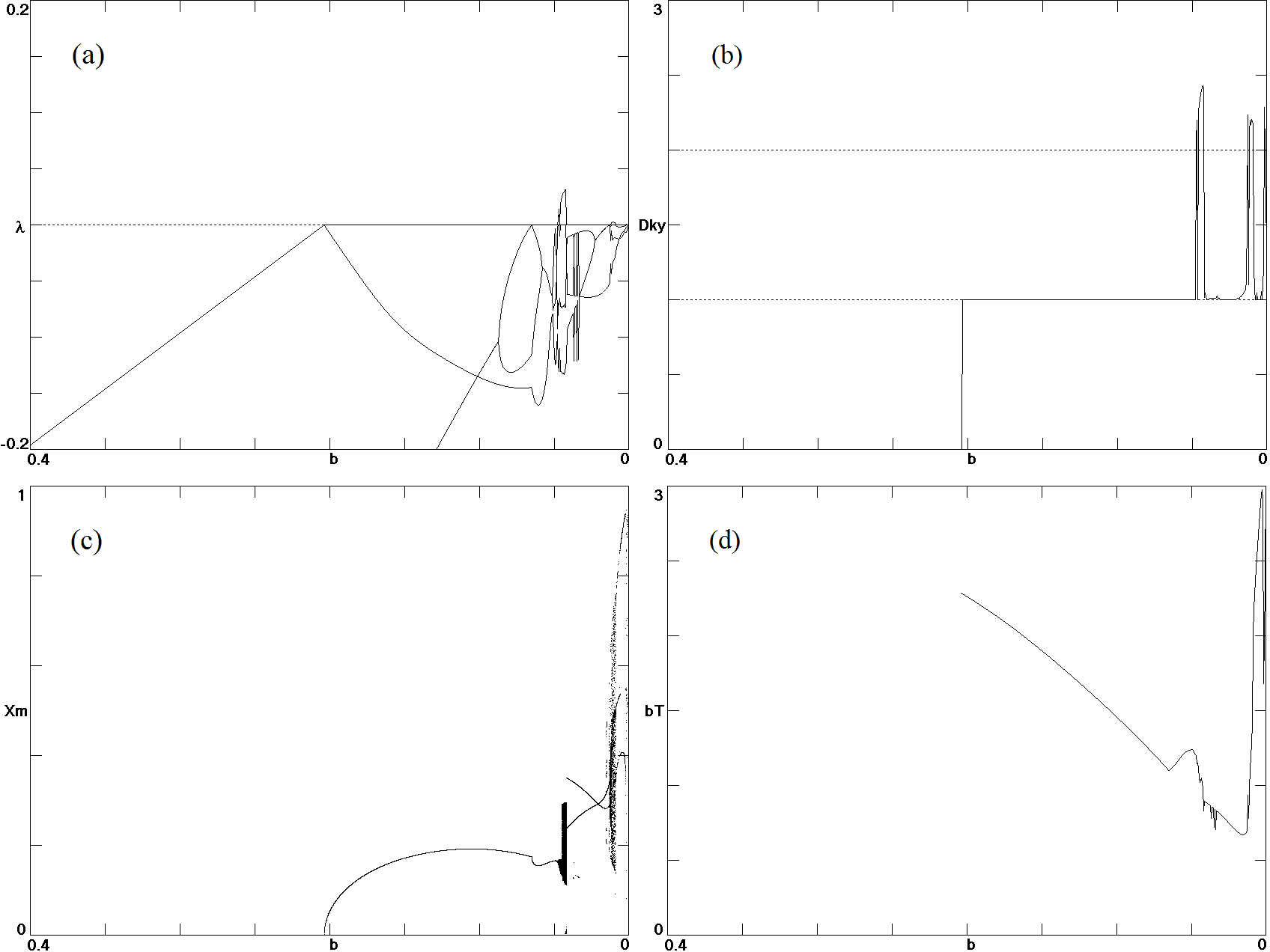
Fig. 7.

Fig. 8.

Fig. 9.
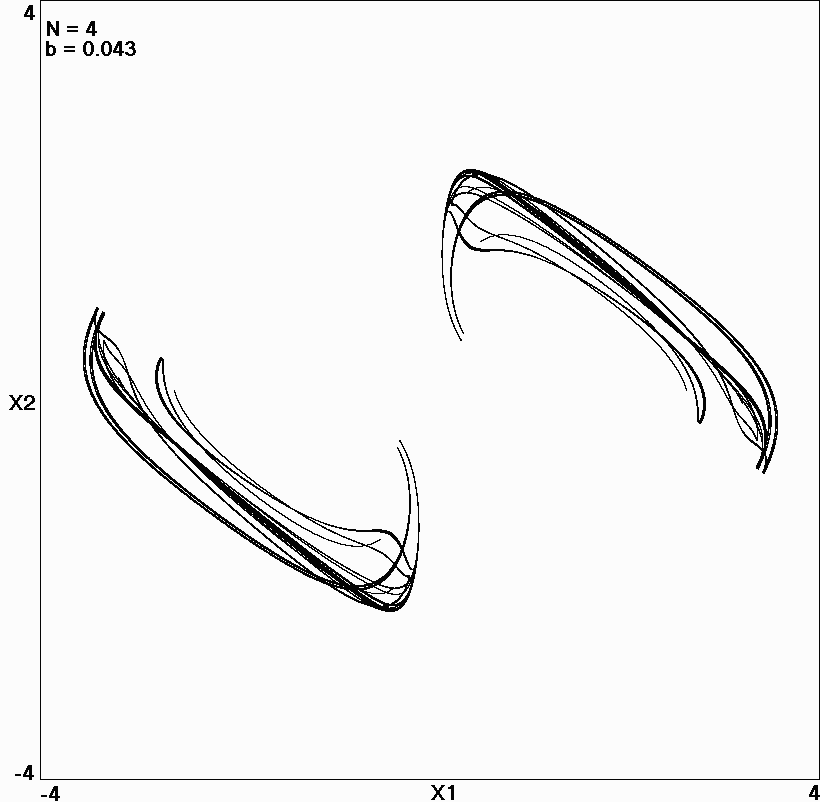
Fig. 10.
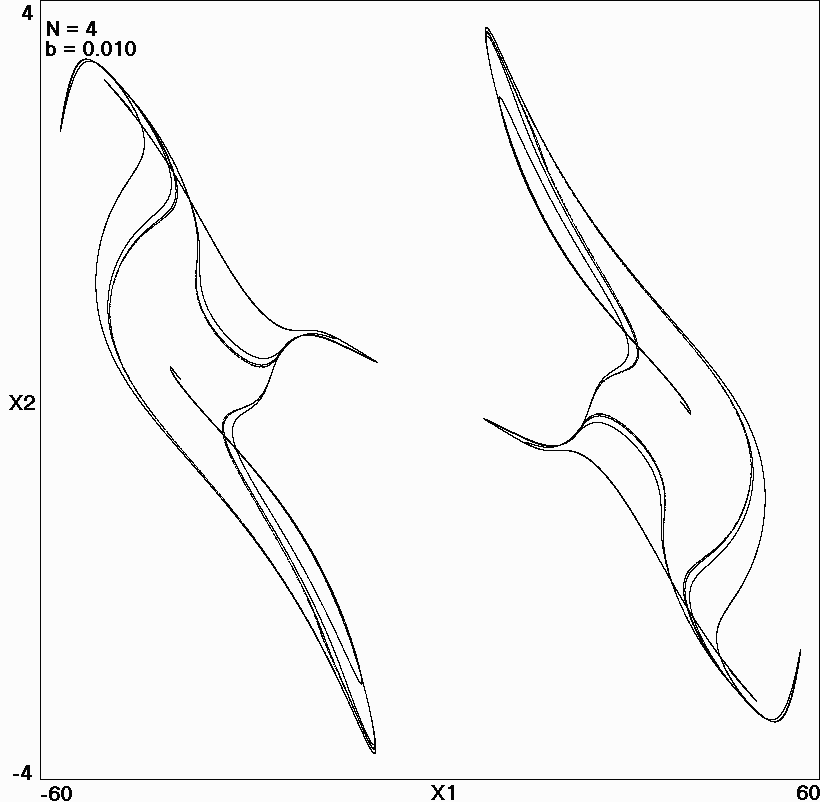
Fig. 11.
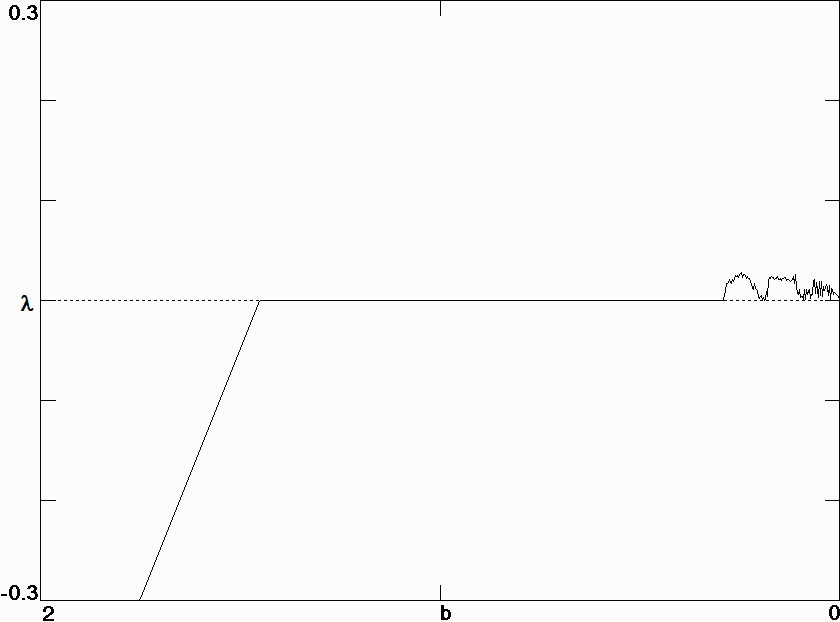
Fig. 12.

Fig. 13.

Fig. 14.

Fig. 15.
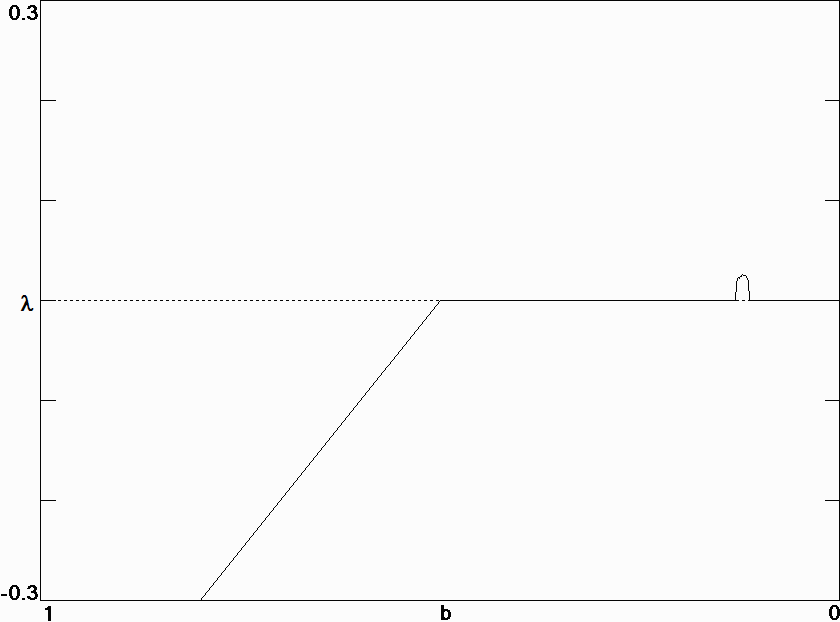
Fig. 16.
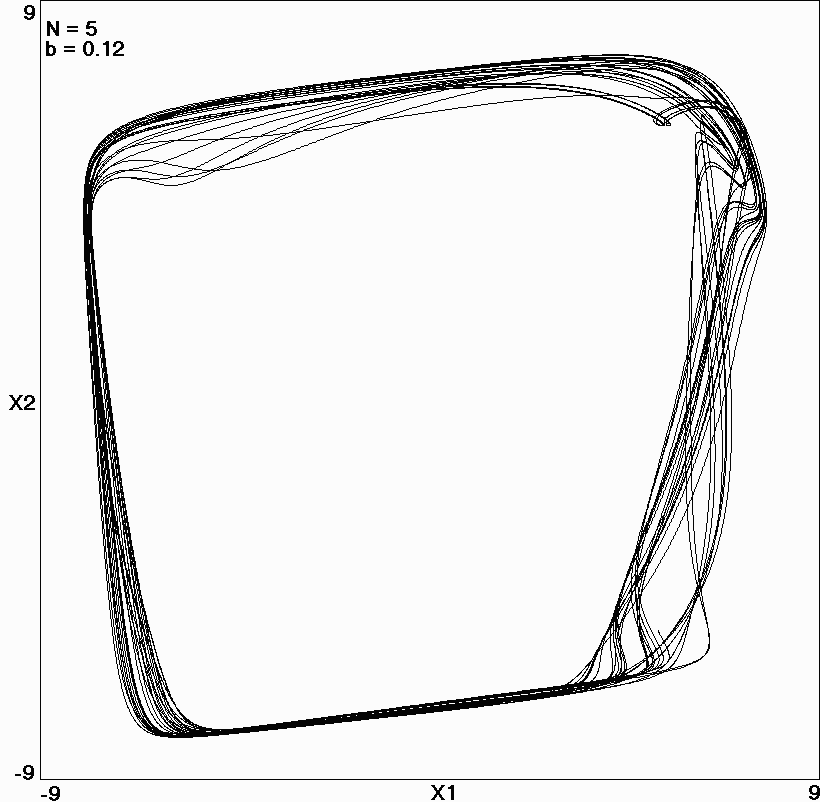
Fig. 17.
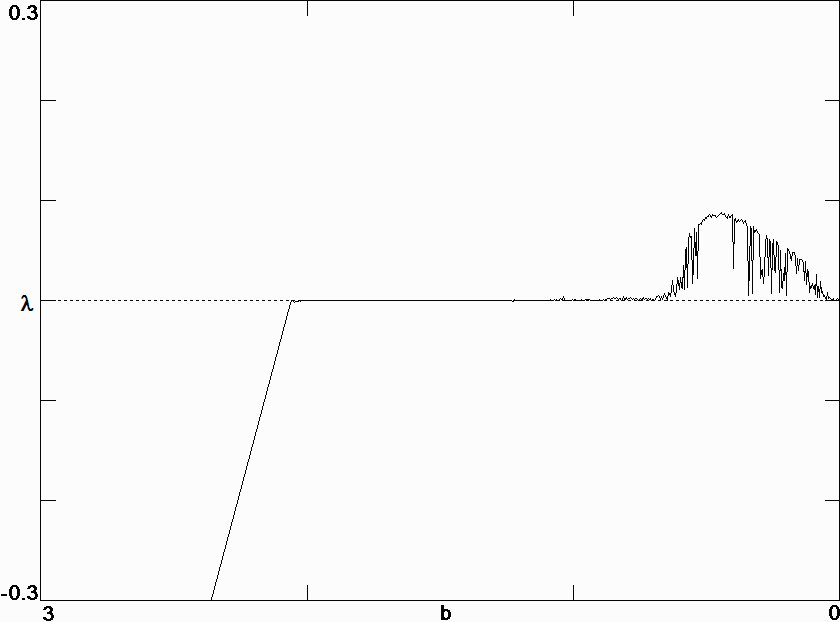
Fig. 18.